Research on Optimal Scale for Extraction of Relief Amplitude in Loess Geological Hazards Assessment Factors: a Case Study of Mizhi County, Yulin City
-
摘要:
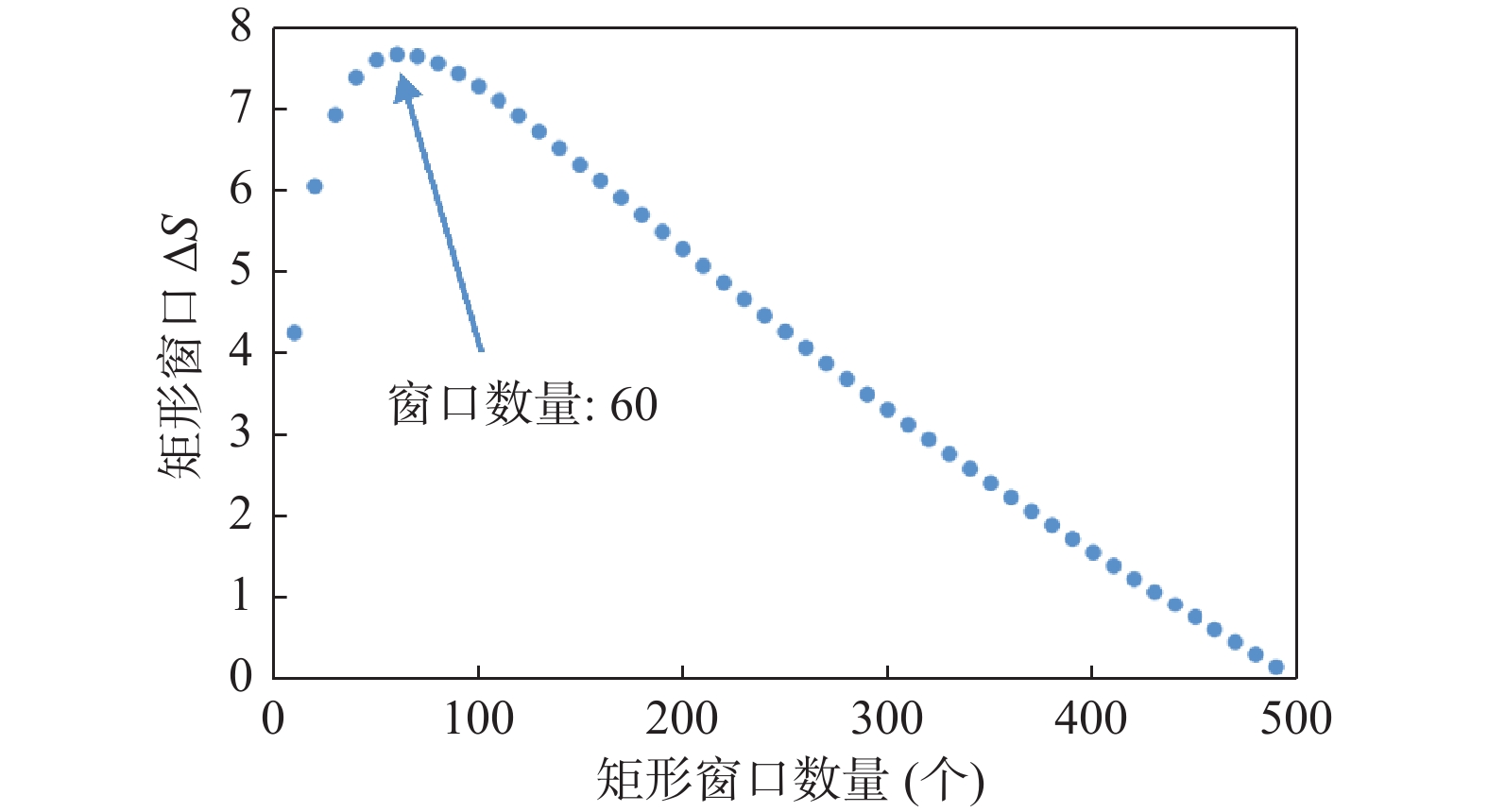
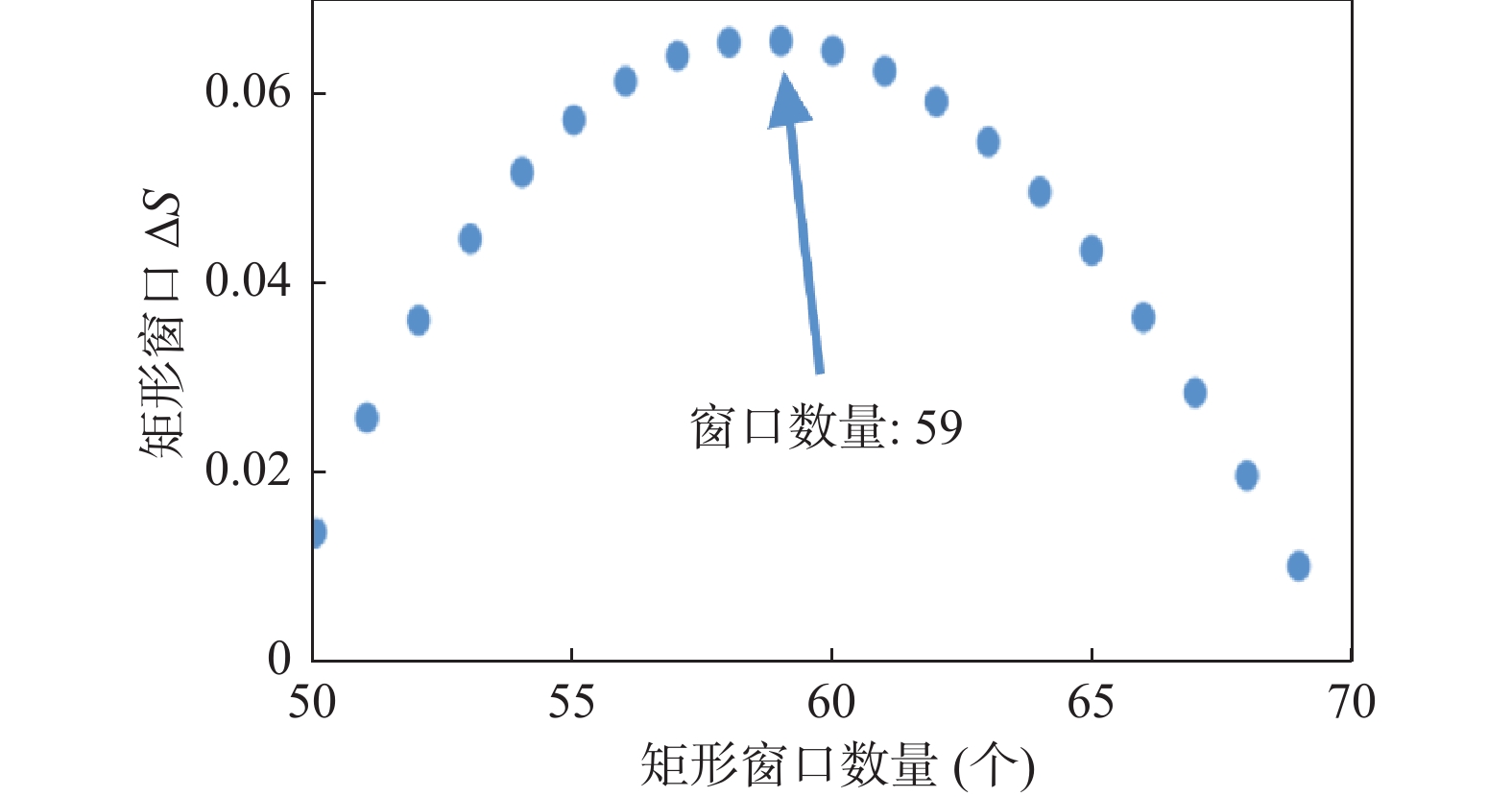
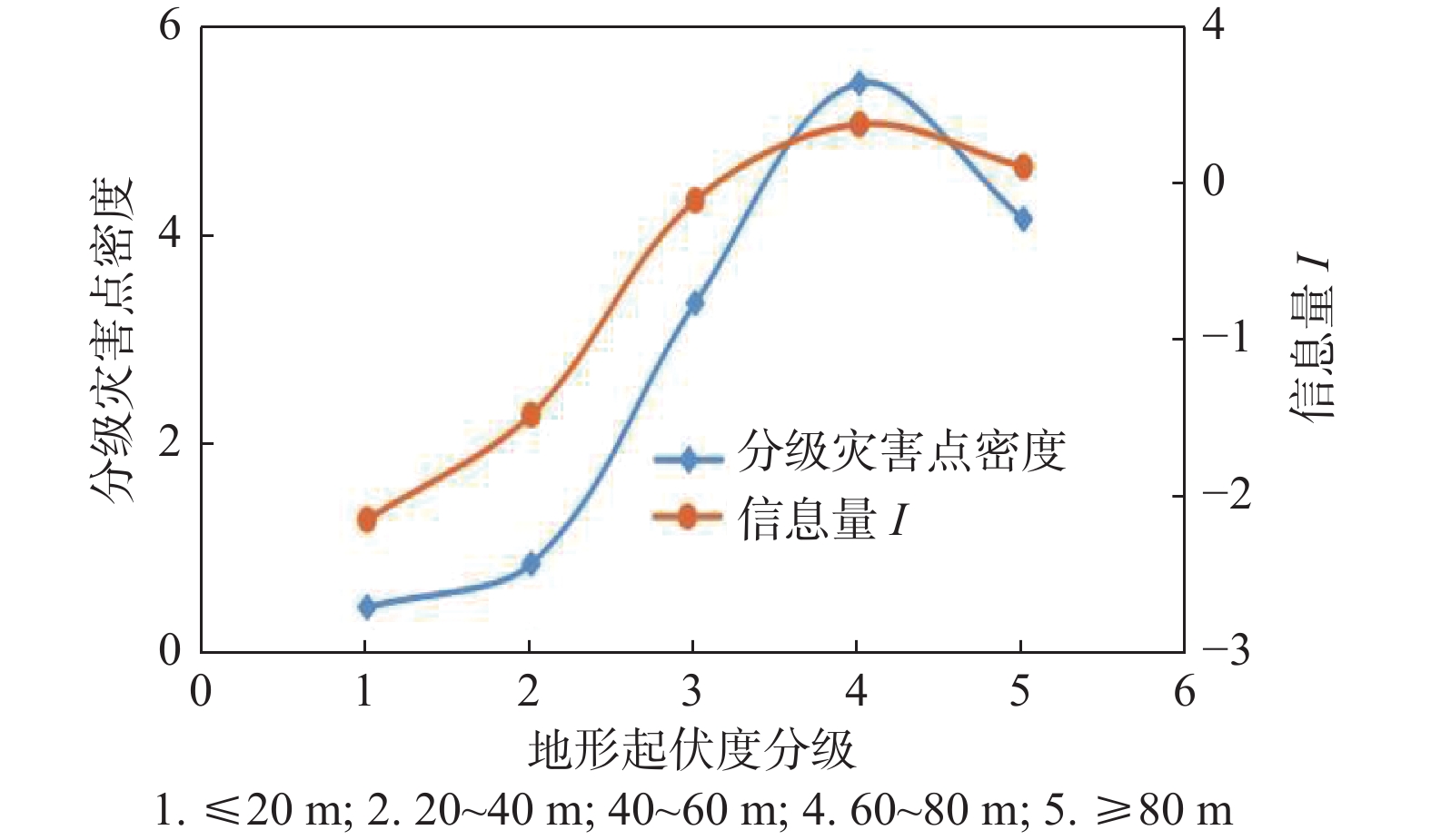
目前地质灾害相关评价工作中，部分研究人员对于“地形起伏度”（也称相对坡高）选取最佳窗口单元进行提取存在着一定程度的随意性和主观性，致使取得的地形起伏度参数与研究区实际情况相比存在一定的误差。后续地灾评价中，无论采用以栅格为基础的信息量模型还是现今普遍流行的各类机器学习方法，其评价因子本身的误差甚至错误会导致评价结果可靠性降低。笔者基于ArcGIS平台，利用陕西省榆林市米脂县分辨率为2 m的DEM数据，采用均值变点分析法，通过两轮分析，数量分别为10×10和1×1的矩形窗口逐渐逼近研究区地形起伏度的最佳统计单元，计算出该县地形起伏度为0～256.60 m，最佳统计单元为59×59的窗格，栅格单元边长为2 m，提取窗格边长为118 m，对应提取面积为13924 m2。随后依据陕北黄土地区历史滑坡及崩塌的易发坡高统计将米脂县地形起伏度等分为＜20 m、20～40 m、40～60 m、60～80 m、＞80 m等5个区间，受原始地形条件及削坡建房、建厂等综合影响，40~80 m为灾害隐患发育的主要区间，灾害隐患点占比为88.60%。结合米脂县地质灾害隐患点信息量值和灾害点密度对比曲线，结果显示二者有很好的相关性，体现了地形起伏度统计单元选取和区间划分的合理性。本研究所采用的高精度DEM数据的计算及分析结果，首先避免了目视寻找拐点的弊端，其次在黄土高原地区千沟万壑的地貌条件中能够满足数字地形分析与精细化地质灾害调查的需求，可为黄土高原区地质灾害评价防治及黄河中上游流域的水土流失治理与生态环境保护提供一定的技术支撑。
Abstract:In the current evaluation of geological hazards, there is a certain degree of arbitrariness and subjectivity in selecting the best window unit scale for the extraction of relief amplitude (also named relative slope height). The certain error exists as compared the obtained relief amplitude parameters with the actual situation in the study area. During the disaster assessment, using grid-based information models or various popular machine learning methods can lead to a reduction in the reliability of the evaluation results due to the errors of the evaluation factors. In the current study, we use DEM data (2 m resolution) of Mizhi County, adopt 10×10, 1×1 rectangular windows for the relief amplitude extraction based on ArcGIS platform, and use the mean change-point analysis to calculate the relief amplitude of Mizhi County from 0–256.60 m, and the best statistical cell is 59×59 with the grid length of 2 m, and the side length of the extraction window is 118m and square is
13924 m2. Afterwards, according to the statistics on the slope height easily inducing historical landslides and collapses in the loess region of northern Shaanxi Province, the relief amplitude in Mizhi County is divided into five intervals: ＜20 m, 20–40 m, 40–60 m, 60–80 m and ＞80 m. Due to the comprehensive influence of the original terrain conditions, slope cutting and building of houses, factories, etc., 40–80 m is the main interval for the development of disaster hazards, with a proportion of 88.60% of disaster hazard points.The comparison curves of information value and hazard point density of each interval were made, which demonstrates the reasonableness of the selection of the statistical unit and the division of the interval of the relief amplitude. The calculation and analysis results of high-precision DEM data adopted first avoid the drawbacks of visually searching for inflection points, and secondly meet the needs of digital terrain analysis and refined geological hazard investigation in the mountainous terrains of the Loess Plateau region. The method and the results can provide technical support for the evaluation and prevention of geological hazards in the Loess Plateau area and the management of soil erosion and ecological environment protection in the middle and upper reaches of the Yellow River basin. -
-
![]()
图 3 矩形窗口提取地形起伏度拟合曲线
Figure 3. Fitting curve of rectangular windows extracting the average reliefamplitude
![]()
图 4 矩形窗口∆S变化曲线（步距10）
Figure 4. Rectangular window difference variation curve (step distance of 10)
![]()
图 5 矩形窗口∆S变化曲线（步距1）
Figure 5. Rectangular window difference variation curve （step distance of 1）
![]()
图 6 米脂县地形起伏度和地质灾害隐患点分布图
Figure 6. Relief amplitude and distribution of geological hazard potential sites in Mizhi County
![]()
图 8 米脂县地形起伏度信息量值与分级灾害点密度对比图
Figure 8. Comparison curve between relief amplitude information quantity value and classified hazard density in Mizhi County
表 1 窗口数量、面积与平均地形起伏度关系统计表
Table 1 Statistics on the number and area of rectangular windows with the average relief amplitude
窗口
数量（个）窗口面积
（m2）平均地形
起伏度（m）窗口
数量（个）窗口面积
（m2）平均地形
起伏度（m）窗口
数量（个）窗口面积
（m2）平均地形
起伏度（m）10 400 12.2912 180 129600 98.6925 350 490000 124.6421 20 1600 24.2089 190 144400 100.8045 360 518400 125.7695 30 3600 34.3080 200 160000 102.8036 370 547600 126.8710 40 6400 42.8923 210 176400 104.7012 380 577600 127.9472 50 10000 50.2548 220 193600 106.5074 390 608400 128.9990 60 14400 56.6414 230 211600 108.2303 400 640000 130.0282 70 19600 62.2494 240 230400 109.8784 410 672400 131.0365 80 25600 67.2303 250 250000 111.4593 420 705600 132.0243 90 32400 71.6998 260 270400 112.9787 430 739600 132.9916 100 40000 75.7451 270 291600 114.4425 440 774400 133.9396 110 48400 79.4344 280 313600 115.8560 450 810000 134.8693 120 57600 82.8203 290 336400 117.2234 460 846400 135.7813 130 67600 85.9471 300 360000 118.5485 470 883600 136.6766 140 78400 88.8487 310 384400 119.8355 480 921600 137.5567 150 90000 91.5523 320 409600 121.0860 490 960400 138.4219 160 102400 94.0813 330 435600 122.3018 500 1000000 139.2725 170 115600 96.4561 340 462400 123.4868 
下载: 导出CSV
表 2 矩形窗口均值变点法统计结果表（步距为10）
Table 2 Statistics results of the mean-change-point method for rectangular window
窗口
数量（个）∆S
（S-Si）窗口
数量（个）∆S
（S-Si）窗口
数量（个）∆S
（S-Si）窗口
数量（个）∆S
（S-Si）10 4.2608 140 6.5372 270 3.8843 400 1.5723 20 6.0529 150 6.3306 280 3.6935 410 1.4080 30 6.9466 160 6.1217 290 3.5051 420 1.2453 40 7.4034 170 5.9119 300 3.3190 430 1.0843 50 7.6176 180 5.7019 310 3.1351 440 0.9249 60 7.6860 190 5.4927 320 2.9534 450 0.7670 70 7.6621 200 5.2846 330 2.7739 460 0.6107 80 7.5773 210 5.0781 340 2.5964 470 0.4559 90 7.4514 220 4.8735 350 2.4210 480 0.3025 100 7.2973 230 4.6711 360 2.2475 490 0.1506 110 7.1236 240 4.4708 370 2.0760 500 120 6.9364 250 4.2729 380 1.9063 130 6.7399 260 4.0774 390 1.7384
下载: 导出CSV
表 3 矩形窗口均值变点法统计结果表（步距为1）
Table 3 Statistics results of the mean-change-point method for rectangular window
窗口
数量（个）窗口
面积（m2）∆S
（S-Si）窗口
数量（个）窗口
面积（m2）∆S
（S-Si）窗口
数量（个）窗口
面积（m2）∆S
（S-Si）50 10000 0.0139 57 12996 0.0642 64 16384 0.0498 51 10404 0.0259 58 13456 0.0656 65 16900 0.0436 52 10816 0.0362 59 13924 0.0657 66 17424 0.0365 53 11236 0.0448 60 14400 0.0647 67 17956 0.0286 54 11664 0.0519 61 14884 0.0626 68 18496 0.0198 55 12100 0.0574 62 15376 0.0593 69 19044 0.0103 56 12544 0.0615 63 15876 0.0551 70 19600
下载: 导出CSV
表 4 地形起伏度分级的地质灾害信息量值
Table 4 The information value base on classification of relief amplitude
地形起伏
度分级分级面积
（km2）灾害点
数量（个）灾害点
百分比分级灾害
点密度信息量I ≤20 m 45.80 20 0.46% 0.4367 − 2.1489 20～40 m 134.80 115 2.62% 0.8531 − 1.4792 40～60 m 494.51 1658 37.82% 3.3528 − 0.1105 60～80 m 407.80 2226 50.78% 5.4586 0.3769 ＞80 m 87.82 365 8.33% 4.1561 0.1043
下载: 导出CSV
-
А•И•斯皮里顿诺夫. 地貌制图学[M]. 北京: 地质出版社, 1956 А•И•СЛИРИДОНОВ. Geomorphological Cartography[M]. Beijing: Geological PublishingHouse, 1956.
陈学兄, 张小军, 常庆瑞, 等. 陕西省地形起伏度最佳计算单元研究[J]. 水土保持通报, 2016, 36(3): 265-270 CHEN Xuexiong, ZHANG Xiaojun, CHANG Qingrui, et al. A Study on Optimal Statistical Unit for ReliefAmplitude of Land Surface in Shaanxi Province[J]. Bulletin of Soil and Water Conservation, 2016, 36(3): 265-270.
封志明, 唐焰, 杨艳昭, 等. 中国地形起伏度及其与人口分布的相关性[J]. 地理学报, 2007, 62(10): 1071-1082. FENG Zhiming, TANG, Yan, YANG Yanzhao, et. al. Relief degree of land surface in China and itscorrelation with population distribution [J]. Acta Geographica Sinica, 2008, 18(2): 237-246.
封志明, 张丹, 杨艳昭, 等. 中国分县地形起伏度及其与人口分布和经济发展的相关性[J]. 吉林大学社会科学学报, 2011, 51(1): 146-160 FENG Zhiming, ZHANG Dan, YANG Yanzhao, et. al. Relief degree of land surface in China at county levelbase on GIS and its correlation between population density and economic development[J]. Jilin UniversityJournal Social Science Edition, 2011, 51(1): 146-160.
郭芳芳, 杨农, 孟晖, 等. 地形起伏度和坡度在区域滑坡灾害评价中的应用[J]. 中国地质, 2008, 35(1): 131-143 GUO Fangfang, YANG Nong, MENG Hui, et al. Application of the relief amplitude and slope analysis toregional landslide hazard assessments[J]. Geology in China, 2008, 35(1): 131-143.
刘新华, 杨勤科, 汤国安. 中国地形起伏度的提取及在水土流失定量评价中的应用[J]. 水土保持通报, 2001, 21(1): 57-59 doi: 10.3969/j.issn.1000-288X.2001.01.015 LIU Xinhua, YANG Qinke, TANG Guoan. Extraction and application of relief of China based on DEM andGIS method[J]. Bulletin of Soil and Water Conservation, 2001, 21(1): 57-59. doi: 10.3969/j.issn.1000-288X.2001.01.015
李双双, 汪成博, 延军平, 等. 面向事件过程的秦岭南北极端降水时空变化特征[J]. 地理学报, 2020, 75(5): 989-1007 LI Shuangshuang, WANG Chengbo, YAN Junping, et. al. Variability of the event-based extreme precipitationin the south and north Qinling Mountains[J]. ACTA GEOGRAPHICA SINICA, 2020, 75(5): 989-1007.
刘元, 韩磊, 等. 基于不同分辨率DEM的黄土高原地形起伏度研究[J]. 河南科技, 2021, 27: 89-94 doi: 10.3969/j.issn.1003-5168.2021.07.036 LIU Yuan, HAN Lei, et. al. Study on Relief Amplitude of the Loess Plateau Based on DEM with DifferentResolutions[J]. Henan Science and Technology, 2021, 27: 89-94. doi: 10.3969/j.issn.1003-5168.2021.07.036
孟庆华. 秦岭山区地质灾害风险评估方法研究—以陕西凤县为例[D]. 北京: 中国地质科学院, 2011: 62 MENG Qinghua. Study on the Methods of Geohazards Risk Assessment in Qinling Mountain—A case studyof Feng County, Baoji City, Shaanxi Province[D]. Beijing: Chinese Academy of Geological Sciences, 2011: 62.
孟晓捷, 张新社, 曾庆铭, 等. 基于加权信息量法的黄土滑坡易发性评价——以1: 5万天水市麦积幅为例[J]. 西北地质, 2022, 55(2): 249-259 MENG Xiaojie, ZHANG Xinshe, ZENG Qingming, et al. The Susceptibility Evaluation of Loess LandslideBased on Weighted Information Value Method—Taking 1: 50000 Map of Maiji District of Tianshui City AsAn Example[J]. Northwestern Geology, 2022, 55(2): 249-259.
宁婷, 崔伟, 马晓勇, 等. 基于均值变点法提取地形起伏度的影响因素分析——以黄河流域(山西段)为例[J]. 测绘通报, 2022, (2): 159-163 NING Ting, CUI Wei, MA Xiaoyong, et al. Analysis of factors affecting the extraction of relief amplitude bymean change point method: taking the Yellow River Basin in Shanxi as an example[J]. Bulletin of SurveyingAnd Mapping, 2022, (2): 159-163.
邱维蓉, 吴帮玉, 潘学树, 等. 几种聚类优化的机器学习方法在灵台县滑坡易发性评价中的应用[J]. 西北地质, 2020, 53(1): 222-233 QIU Weirong, WU Bangyu, PAN Xueshu, et al. Application of Several Cluster-optimization-based MachineLearning Methods in Evaluation of Landslide Susceptibility in Lingtai County[J]. Northwestern Geology, 2020, 53(1): 222-233.
宿星, 魏万鸿, 郭万钦, 等. 基于SRTM DEM的地形起伏度对天水市黄土滑坡的影响分析[J]. 冰川冻土, 2017, 39(3): 616-622 SU Xing, WEI Wanhong, GUO Wanqin, et. al. Analyzing the impact of relief amplitude to loess landslidesbased on SRTM DEM in Tianshui Prefecture[J]. Journal of Glaciology and Geocryology, 2017, 39(3): 616-622.
孙萍萍, 张茂省, 程秀娟, 等. 黄土高原地质灾害发生规律[J]. 山地学报, 2019, 3 7(5): 737-746 SUN Pingping, ZHANG Maosheng, CHENG Xiujuan, et. al. On the Regularity of Geological Hazards on theLoess Plateau in China[J]. Mountain Research. 2019, 3 7(5): 737-746.
孙萍萍, 张茂省, 贾俊, 等. 中国西部黄土区地质灾害调查研究进展[J]. 西北地质, 2022, 55(3): 96-107. SUN Pingping, ZHANG Maosheng, JIA Jun, et. al. Geo- hazards research and investigation in the LoessRegions of Western China[J]. Northwestern Geology, 2022, 55(3): 96- 107.
唐亚明, 张茂省, 李政国, 等. 国内外地质灾害风险管理对比及评述[J]. 西北地质, 2015, 48(2): 238-246 doi: 10.3969/j.issn.1009-6248.2015.02.025 TANG Yaming, ZHANG Maosheng, Li Zhengguo, et. al. Review and Comparison onInland and Overseas Geo-hazards Risk Management[J]. Northwestern Geology, 2015, 48(2): 238-246. doi: 10.3969/j.issn.1009-6248.2015.02.025
涂汉明, 刘振东, 等. 中国地势起伏度最佳统计单元的求证[J]. 湖南大学学报(自然科学版), 1990, 12(3): 266-271 TU Hanming, LIU Zhendong, et. al. Demonstrating on optimum statistic unit of relief amplitude in China[J]. Journal of Hubei University(Natural Science), 1990, 12(3): 266-271.
王让虎, 张树文, 蒲罗曼, 等. 基于ASTERGDEM和均值变点分析的中国东北地形起伏度研究[J]. 干旱区资源与环境, 2016, 30(6): 49-54 WANG Ranghu, ZHANG Shuwen, PU Luoman, et al. Analysis on the relief amplitude in northeast ChinaBased on ASTER GDEM and mean change point method[J]. Journal of Arid Land Resources andEnvironment, 2016, 30(6): 49-54.
王志恒, 胡卓玮, 赵文吉, 等. 应用累积和分析算法的地形起伏度最佳统计单元确定[J]. 测绘科学, 2014, 39(6): 59-64 doi: 10.16251/j.cnki.1009-2307.2014.06.004 WANG Zhiheng, HU Zhuowei, ZHAO Wenji, et al. Extracting optimum statistical unit for relief degree ofland surface with CUSUM algorithm[J]. Science of Surveying and Mapping, 2014, 39(6): 59-64. doi: 10.16251/j.cnki.1009-2307.2014.06.004
吴常润, 赵冬梅, 刘澄静, 等. 基于GIS和信息量模型的陇川县滑坡易发性评价[J]. 西北地质, 2020, 53(2): 308-320 WU Changrun, ZHAO Dongmei, LIU Chengjing, et al. Landslide Susceptibility Assessment of LongchuanCounty Based on GIS and Information Value Model[J]. Northwestern Geology, 2020, 53(2): 308-320.
伍剑波, 孙强, 张泰丽, 等. 地形起伏度与滑坡发育的相关性——以丽水市滑坡为例[J]. 华东地质, 2022, 43(2): 235-244 WU Jianbo, SUN Qiang, ZHANG Taili, et. al. Research for the correlation between relief amplitude andlandslides: a case study of Lishui City[J]. East China Geology, 2022, 43(2): 235-244.
薛强, 张茂省, 董英, 等. 基于DEM和遥感的黄土地质灾害精细化风险识别——以陕北黄土高原区米脂县为例[J]. 中国地质, 2023, 50(3): 907-923 XUE Qiang, ZHANG Maosheng DONG Ying, et. al. Refinement risk identification of loess geo-hazardsbased on DEM and remote sensing——Taking Mizhi County in the Loess Plateau of Northern Shaanxi as anexample[J]. Geology in China(in Chinese with English abstract), 50(3): 907-923.
游珍, 封志明, 杨艳昭, 等. 中国1km地形起伏度数据集[J]. 全球变化数据学报, 2018, 2(2): 151-155 YOU Zhen, FENG Zhiming, YANG Yanzhao, et. al. Relief Degree of Land Surface Dataset ofChina(1km)[J]. Journal of Global Change Data & Discovery, 2018, 2(2): 151-155.
张茂省, 薛强, 贾俊, 等. 地质灾害风险管理理论方法与实践[M]. 北京: 科学出版社, 2021 ZHANG Maosheng, Xue Qiang, Jia Jun, et. al. Theory, Method and Practice of Geological Hazard RiskManagement[M]. Beijing: Science Press, 2021.
张锦明, 游雄. 地形起伏度最佳分析区域研究[J]. 测绘科学技术学报, 2011, 28(5): 369-373 doi: 10.3969/j.issn.1673-6338.2011.05.014 ZHANG Jinming, YOU Xiong. Investigating Optimum Statistical Unit of Relief[J]. Journal ofGeomatics Science and Technology, 2011, 28(5): 369-373. doi: 10.3969/j.issn.1673-6338.2011.05.014
张明媚, 薛永安. 斜坡地质灾害敏感性评价中地势起伏度提取最佳尺度研究[J]. 太原理工大学学报, 2020, 51(6): 881-888 ZHANG Mingmei, XUE Yongan. Optimal Scale for Extracting Relief Amplitude in Slope GeologicalHazard Sensitivity Evaluation[J]. Journal of taiyuan university of technology, 2020, 51(6): 881-888.
张志沛, 魏在豪, 等. 基于加权信息量模型的滑坡灾害易发性评价——以灞桥区为例[J]. 科学技术与工程, 2020, 20(9): 3492-3500 doi: 10.3969/j.issn.1671-1815.2020.09.018 ZHANG Zhipei, WEI Zaihao, et al. Landslide Susceptibility Assessment Based on Weighted InformationValues Model: Take Baqiao District as an Example[J]. Science Technology and Engineering, 2020, 20(9): 3492-3500. doi: 10.3969/j.issn.1671-1815.2020.09.018
赵斌滨, 程永锋, 丁士君, 等. 基于SRTM-DEM的中国地势起伏度统计单元研究[J]. 水利学报, 2015, (S1): 284-290 ZHAO Binbin, CHENG Yongfeng, DING Shijun, et al. Statistical unit of relief amplitude in China based onSRTM-DEM[J]. Shuili Xuebao, 2015, (Sl): 284-290.
朱红春, 陈楠, 刘海英, 等. 自1: 10000比例尺DEM提取地形起伏度——以陕北黄土高原的实验为例[J]. 测绘科学, 2005, 30(4): 86-88 doi: 10.3771/j.issn.1009-2307.2005.04.028 ZHU Hongchun, CHEN Nan, LIU Haiying, et. al. Research on the relief based on 1: 10000 DEMs--A casestudy in the loess plateau of north Shaanxi province[J]. Science of Surveying and Mapping, 2005, 30(4): 86-88. doi: 10.3771/j.issn.1009-2307.2005.04.028
Ghimire M. Geo-hydrological hazard and risk zonation of Banganga watershed using GIS and remote sensing[J]. Journal of Nepal Geological Society, 2001, 23: 99-110.
Shannon C E. A mathematical theory of communication[J]. Bell Labs Technical Journal, 1948, 27(4): 623-656. doi: 10.1002/j.1538-7305.1948.tb00917.x
计量
- 文章访问数: 196
- HTML全文浏览量: 23
- PDF下载量: 53
